Dynamics of boundary sandpile

Problem's Description

Let $\mu_0$ be a given (mass) distribution on $\mathbb Z^d$, i.e. a non-negative function supported on finitely many sites of $\mathbb Z^d$. Fix also a threshold $\kappa_0>0$. For each integer $k\geq 0$ we inductively construct a sequence of sets $V_k$, mass distributions $\mu_k$, and functions $u_k$ as follows. Start with $V_0 = \mathrm{supp} \mu_0$ and $u_0=0$. For an integer time $k\geq 0$ a particular site $x\in \mathbb Z^d$ is called unstable if either of the following holds:

(a) $x\in \partial V_k$ and $\mu_k (x) > \kappa_0$,

(b) $x\in \, \overset{\circ}{V_k}$ and $\mu_k (x) >0$.

Otherwise a site is called stable. We call the number $\kappa_0$ the (boundary) capacity of the model and refer to $V_k$ as the set of visited sites at time $k$.

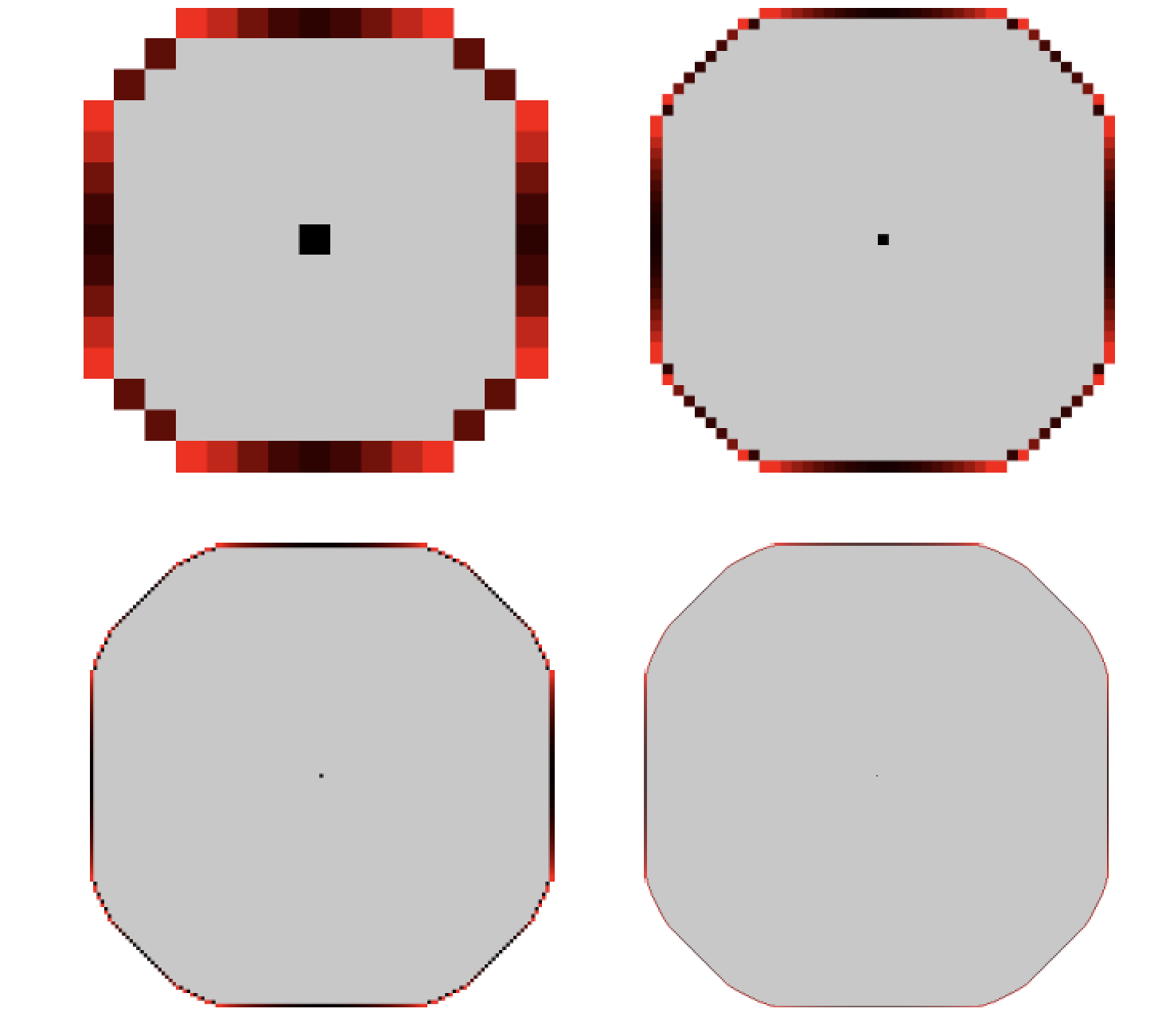
Any unstable site can topple by distributing all its mass equally among its $2d$ lattice neighbours. More precisely, for each $k\geq 0$ we choose an unstable site $x\in V_k$ and define $V_{k+1} = V_k \cup \{y\in \mathbb Z^d: \ y\sim x \}$, \begin{equation} \tag{1} \mu_{k+1}(y) = \begin{cases} 0, &\text{if $y=x$}, \\ \mu_k(y) + \frac{1}{2d}\mu_k(x) ,&\text{if $ y\sim x $}, \\ \mu_k(y), &\text{otherwise}, \end{cases} \end{equation} and $u_{k+1} (y) = u_k(y) + \mu_k(y) \delta_0(y-x)$, $y\in \mathbb Z^d$, where $\delta_0$ is the Kronecker delta symbol at the origin, i.e. $\delta_0(x)$ equals 1 if $x=0$ and is zero otherwise for $x\in \mathbb Z^d$. We call $u_k$ the odometer function at time $k$. For the sake of convenience, we do allow the toppling to be applied to a stable site, as an identity operator, i.e. if at time $k$ a toppling is applied to a stable site $x$, then we set $V_{k+1}= V_k$, $u_{k+1}=u_k$ and $\mu_{k+1}=\mu_k$. We say that toppling $x$ is legal, if $x$ is unstable. If for some $k$ there are no unstable sites, the process is terminated. We call this model boundary sandpile (BS) and denote by $BS(\mu_0, \kappa_0)$, where $\mu_0$ is the initial distribution, and $\kappa_0$ is the boundary capacity of the model.

It is clear that the triple $(V_k, \mu_k, u_k)_{k=1}^\infty$ may depend on the choice of the unstable sites, i.e. the toppling sequence. Later on we will see that for a suitable class of toppling sequences stable configurations exist and are identical (see Propositions 2.1, 2.3 in [1]). Observe that from the definition of discrete Laplacian and (1) above, we easily see that for each $k\geq 0$ one has \begin{equation} \Delta u_k (x) = \mu_k(x) - \mu_0(x) , \qquad x\in \mathbb Z^d, \end{equation} i.e. the Laplacian of $u_k$ represents the net gain of mass for a site $x$ at time $k$.

1. ArticleIs an originDiscrete Balayage and Boundary Sandpile

   • Hayk Aleksanyan,
   • Henrik Shahgholian

   Journal d'Analyse Mathématique, 2017arXiv

2. Article Perturbed divisible sandpiles and quadrature surfaces

   • Hayk Aleksanyan,
   • Henrik Shahgholian

   pp. 1-29, year of publication: 2017arXiv

3. Thesis Limit Theorems for Internal Aggregation Models,

   • Lionel Levine

   PhD thesis, University of California Berkley, 2007

4. Article Strong spherical asymptotics for rotor-router aggregation and the divisible sandpile

   • Lionel Levine,
   • Yuval Peres

   Potential Anal. 30 (1), 1-27, 2009arXiv

1. Open Scaling limits of BS

2. Open The limiting PDE of BS

3. Open Other type of processes

4. Open Random walks governed by BS