Convexity of Abelian Sandpile

General Description View the group

Abelian Sandpile Model (ASM) is a lattice growth model for configurations of chips distributed on vertices of $\mathbb Z^d$. A vertex carrying at least $2d$ chips topples giving a single chip to all its $2d$ lattice neighbours, and losing $2d$ chips itself. If there are no sites with more than $2d-1$ chips, the process terminates. For any finite non-negative initial configuration of chips, subsequently toppling all sites with at least $2d$ chips, the process terminates in finite steps. This process is abelian in the sense that the final configuration of chips is independent of the order of toppling. The model originates in the work of Bak-Tang-Wiesenfeld [2].

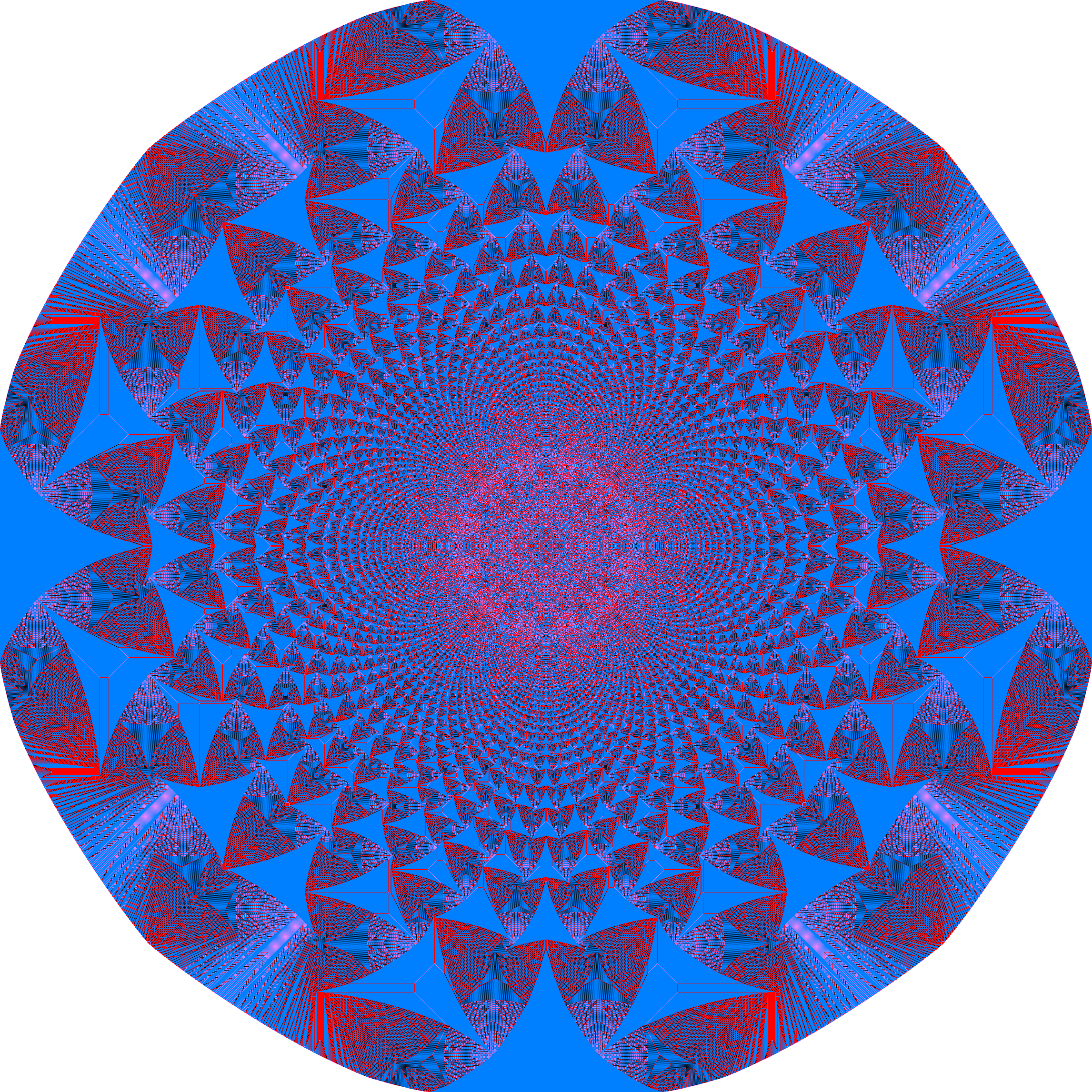
As the number of particles tend to infinity the ASM has a uniques scaling limit which tends to a free boundary problems with facets. See [3].

Problem's Description

Problem: Is the scaling limit of ASM convex?

In [1] the authors prove that in certain directions ASM is convex, and that the boundary of ASM is Lipschitz graph locally. It seems that a complete convexity proof has to use further properties of the model, either in its combinatorial setting or the scaling limit setting in terms of viscosity solutions to certain free boundary problems.

1. ArticleIs an originDiscrete Balayage and Boundary Sandpile

   • Hayk Aleksanyan,
   • Henrik Shahgholian

   Journal d'Analyse Mathématique, 2017arXiv

2. ArticleIs an originSelf-organized criticality: An explanation of the 1/f noise

   • Per Bak,
   • Chao Tang,
   • Kurt Wiesenfeld

   Physical Review Letters 59, 381, 1987

3. ArticleIs an originConvergence of the Abelian sandpile

   • Wesley Pegden,
   • Charles K Smart

   Duke Mathematical Journal 162, 627-642, 2013